Laws of Friction, with Applications to Motorcycling
First Law of Friction
We all think we know how friction works. But pick up a physics book such as Fundamentals of Physics by Halliday, Resnick, and Walker, or a book such as Friction by Bowden and Tabor, and read the two laws of friction and you'll get a surprise. The first law is easy to believe: The friction between two surfaces is proportional to the force pressing one to the other. This force could be the weight of a motorcycle pressing the tire into the pavement, or the clamping force pressing two pieces of wood together. "Proportional" just means that if you double the pressing force you double the friction.The second law is where all the trouble starts. To understand it, suppose you set up an experiment. You put a brick on a table and investigate how much force it takes to start the brick sliding. You screw an eyebolt into the brick, run a line from the eyebolt to a pulley on the edge of the table, and then attach weights to the end of the line. You add weight until the brick starts to slide.
Second Law of Friction
Now here's the interesting part, and the surprising part. You would notice that the orientation of the brick doesn't make any difference. That is, the friction is the same whether the brick is on its large face, the smaller side, or the small end. The friction is independent of the contact area.Don't believe it? You're not alone. Bowden and Tabor, in their book Friction , tell about one of the first modern investigators of friction, Guillaume Amontons. In 1699 Amontons published a paper on friction in which he reported on the two laws. As Bowden and Tabor put it, "The second law, that friction is independent of the size of the bodies, was viewed by the [French Royal Academy of Sciences] with astonishment and skepticism. They instructed their senior academician De la Hire (1640-1718) to repeat Amontons' experiments and check their validity. This he did and confirmed Amontons' conclusions. Amontons' laws of friction have remained with us to this day as a very good working approximation."
I still don't believe it.
It'd probably be less counterintuitive if we could see the microscopic texture of surfaces. Imagine a flat perfectly smooth metal plate. It's flat and perfectly smooth, right? If you think so, you've never seen a micrograph of a flat perfectly smooth plate. It's actually scratched and pitted, like a mountain range in the small.Now imagine a couple of mountain ranges on plates. Turn one over and put it onto the other. The actual contacts will be parts of the various peaks of the two plates onto whatever part of the other plate happens to match the peak. Those peaks — asperities, the tribologists call em — are where the friction occurs, not, obviously, in the valleys where there is no contact. Press em harder and you'd get more actual contact as the asperities bend under the pressure.
If you take another pair of mountain ranges/plates of twice the area and pressed them together with the same pressure, the actual contact area of asperities wouldn't change. You'd have the same number of asperities in contact, but further apart, in the larger plates. Only additional pressure would change the true contact area.
That, in a nutshell, is the model that people use to make sense of the experimental fact that F=μN: friction F is proportional to the force N pressing two surfaces together, with proportionality constant μ. (Apparent) area of contact does not enter into it.
Does this really apply to rubber?
Sometimes people object that the above model may be satisfactory for rigid materials like metals, but rubber would flow into the valleys and you'd get better contact. They miss the fact that rubber at the microscopic scale is quite as irregular as metals are, and that rigid materials aren't rigid at the microscopic level. Pressing rubber onto an irregular surface such as pavement will indeed result in greater area of actual contact (as opposed to the apparent macroscopic area) than might be the case with a metal on pavement, but that contact is still between irregular surfaces; there will still be plenty of gaps in contact. Reducing the gaps can be achieved by pressing harder, which will result in more friction — just as the equation F=μN predicts.Nevertheless, people have been writing me for years with the claim that rubber friction does depend on contact area. They can be quite obnoxious about it. A recent contact was so obnoxious that I repeated the high-school physics experiment, just to be sure. You can read about it here. The bottom line: To a good approximation, rubber friction is governed by the same F=μN which describes friction of more obviously solid materials, and like them is independent of apparent contact area.
Tire load sensitivity
A reader, Adam Lilley, was kind enough to point out a factor I had missed (and did it courteously!), namely tire load sensitivity. A search led to some information from the Society of Automotive Engineers (SAE) stating that the coefficient of friction decreases slightly as load on the tire increases. Classical theory says that the coefficient of friction is constant over a very wide range of loads.What this means is that, for instance, if you double the vertical load on a tire -- that is, you double the weight -- classical theory predicts the traction would also double. But in fact the increase is less than double.
This does not affect the independence of contact area. The load on a tire is the force pressing it into the pavement or other surface. It is a force, not a pressure (which is force per area). So the force on a skinny tire might be, say, 250 pounds for some application. If you change to a fat tire, the force would be unchanged, 250 pounds. Thus the traction will be unchanged.
Application of the First Law
With this information and no more, we can understand two important facets of motorcycle operation. The first law, that friction (which we call traction in the context of tires on road) increases as weight increases, explains why the front brake on a motorcycle (and a car for that matter) is so much more effective than the rear brake. As you slow a vehicle, the weight transfers to the front. We all recognize this; it's why the bag of groceries on the seat slides onto the floor when you get hard on the brakes. So the front tire is now carrying more load than it was; thus it now has more traction than it did. The opposite is happening at the rear: The rear tire is now lighter than it was, and so has less traction. The harder you brake the more the weight and hence the traction transfers forward.Application of the Second Law
Everyone knows that you have less traction in a lean than when the motorcycle is straight up and down. This is because, in a lean, there's less rubber on the road.Right? Well, if you've been paying attention, you'll realize that what everyone knows, is wrong. The second law says that friction is independent of contact area. That implies that, if the traction is indeed less, it isn't because there's less rubber on the road. The second law says that the amount of rubber on the road is not relevant. (It's interesting to me that people who know what everyone knows always overlook the fact that there isn't less rubber on the road in a lean. Motorcycle tires are round. The amount of rubber on the road is the same whether the motorcycle is straight up and down or leaned over.)
So what everyone knows about why there's less traction in a lean, is wrong. Maybe everyone is wrong about the reduced traction as well? They are. The first law says that the friction between two surfaces is dependent only on the force pressing them together; in our application, that's the weight of the motorcycle. Does the motorcycle weigh less in a lean than straight up and down? Of course not; if that were true, we could lose weight simply by leaning over.
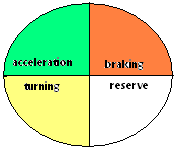 |
Ok, so we've concluded that the amount of traction in a lean is exactly the same as when straight. So what's the origin of this myth? It's certainly true that if I'm leaned far over and apply much braking I'll lose traction and slide. What's the deal, if there's just as much traction in a lean as straight? The explanation is that one's available traction has to be shared between the various users of traction. The Motorcycle Safety Foundation has a good image in their experienced rider course book. The total traction available is represented as an oval or circle, and the things which use traction are partitioned in it. |
| So with this in mind, it's not hard to understand why we can't brake hard while in a lean. When cornering hard, most of our traction is being used for turning, with some for acceleration, and little or none for braking. The diagram looks like this: |  |
The size of the circle is the same because we have the same amount of traction as we did while straight, just as the first law requires. Much of our traction is used in cornering. There's some used in accelerating, if we're on the gas through the curve. Drag at the wheels is always present so I've represented a small amount of traction for that. So what's left over, the white "reserve" sliver, represents all the traction that we have to work with for any emergency, such as braking for an unexpected obstacle or because we went into the corner too fast. It is the small size of the reserve which gives rise to the myth that traction is lacking in curves. Or, if you prefer, it isn't a myth, merely worded differently. The traction which one has to work with in curves is much smaller than that available in a straight line.
Objection!
So if traction doesn't depend on amount of rubber, how come high-performance tires are so large? Hunh?To understand this, we have to make the first law more precise. The friction between two surfaces is proportional to the force pressing them together. But the friction between these two surfaces can be quite different from the friction between these other two surfaces, even if the force on them is the same. It's easier to slide a steel plate on pavement than a rubber tire on pavement, even when both are loaded with, say, 5 pounds.
The two laws can be combined into a single equation: F = μW, where F is the friction between two given surfaces, W is the force pressing them together (the weight, in most of our examples), and μ (the Greek letter mu) is a number called the coefficient of friction. This equation says that the friction is a percentage of the total weight on two surfaces, the percentage being given by the coefficient of friction. (That's really just the first law, saying that the friction is proportional to the weight, the constant of proportionality being given by μ. The second law is incorporated by the fact that the area of contact does not appear in the equation.)
The fact that some surfaces are "stickier" than others is reflected in the different coefficients of friction. Steel on pavement has a much lower value of μ than rubber on pavement. And what's more, different rubber compounds have different values of μ. You want more traction? Just use stickier rubber in your tires. A further complication is that the coefficient of friction varies with various physical parameters. For instance, cold rubber is harder and thus less sticky than warm rubber; the same equation applies but μ is lower for the cold rubber. Static friction, the force necessary to start sliding, is greater than the friction created while sliding. In riding, we're concerned with rolling friction, which is somewhere between static and sliding friction. Again, same equation, but three different values of μ.
If you switch to tires with a better coefficient of friction, you'll immediately notice that stickier rubber is also softer; it wears out much more quickly. Motorcyclists accept the need to replace high-performance tires more often than touring-oriented rubber, but the auto types have another option: They can use wider tires to spread the wear over more rubber. And there, finally, is the reason high-performance auto tires are wider. It isn't to get better traction. Better traction comes from stickier rubber. The tires are wider to get acceptable wear from the stickier, softer rubber. (As well as for other things like heat management, rigidity under the stresses of cornering and braking and acceleration, etc.)
So you admit there are effects on traction due to contact patch area?
Yes, absolutely. Consider a skinny little bicycle tire of the same rubber as your 180-section or 190-section sportbike rear tire. Same rubber, so same coefficient of friction, so same resistance to sliding — until you apply the 1000cc power. Then the skinny tire immediately tears in half because it doesn't have the bulk strength of the big tire. If you apply power gently, to avoid ripping it up, you'll be ok — until you try to corner hard, when the same problem happens for the same reason. If you try to avoid that problem by riding in a straight line, you'll be ok — until the tire overheats and gets slippery because it can't absorb and dissipate heat like the big tire does. Anyone who's hit a small rock, even a pebble, while leaned on a bicycle will know that the small tire will be pushed around a lot more than the tire with the larger contact area. But on the plus side, the skinny tire will probably hydroplane a lot less than the bigger tire.So I'm not saying that you can replace your huge tires with tiny tires. Other things being equal, contact area doesn't change resistance to sliding. If you change contact area so much that other things are no longer comparable — like the bulk strength, heat management, and stability in the previous example — then the handling can be much different. I want to understand the various factors involved so that when I'm considering a change such as fitting a 190-section rear tire on my Bandit with original 180-section rear tire, or putting an automobile tire on a bike in place of a motorcycle tire, what can I expect to happen? And with changes such as those, you will probably not see a significant difference in traction, and certainly not due to any change in contact area.
